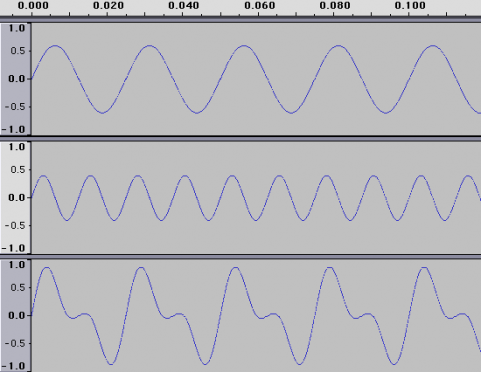
Additive Synthesis
Emulates timbre (tone color) by combining numerous waveforms pitched to different harmonics.
Requires multiple oscillators for each note.
Examples: Pipe and tone wheel organs, Synclavier (Early synthesizer and sampler c. 1970s).
In the above picture, the first two sine waves are combined or "added" together to produce a third, different wave.
Subtractive Synthesis
Whereas additive synthesis is the process of combining individual partials (harmonics) to construct a complex sound, subtractive synthesis is essentially the reverse of this process.
By starting with a harmonically (or partially) rich sound, a subtractive system will filter and modify the signal to reduce it to a desired form.
By doing this, one can use one or two oscillators instead of a bank of 10 to achieve the similar sonic results.
Subtractive synthesis is an extremely popular method of synthesis that has been employed in hardware and software synthesizers since their popularity skyrocketed in the 1970's.
This method of synthesis can be found traditionally in old modular or compact analogue synthesizers as well as modern virtual analogue models and software synthesizers.
Frequency Modulation/FM Synthesis

The frequency of a primary waveform (carrier) is modulated by a secondary waveform (modulator) resulting in changes in timbre.
From Tutorial for Frequency Modulation Synthesis:
When the frequency of the modulator (M) is in the sub-audio range (1-20 Hz), we can hear siren-like changes in pitch of the carrier (C).
However, when we raise M [into the audible] range (above 30 Hz) then we hear a new timbre composed of frequencies called sidebands.
To determine which sidebands are present, we have to control the ratio between the carrier frequency (C) and the modulating frequency (M).
Yamaha DX7 is the biggest example of FM synthesis
Granular Synthesis
Is a sound synthesis method for digital music instruments (synths) that operates on the microsound time scale.
It is often based on the same principles as digital sampling but often includes analog technology.
Instead of a set of sinewaves whose frequencies and amplitudes change over time, graintable synthesis uses many thousands of very short samples split into small pieces (around 1 to 50 ms in length) of overlapping sound bursts or grains.
Multiple grains may be layered on top of each other all playing at different speeds, phase and volume.
The result is no single tone, but a sound scape that is subject to manipulation in a way unlike any natural sound and also unlike the sounds produce by most other synthesis techniques.
By varying the waveform, envelope, duration, spatial position, and density of the grains many different sounds can be produced.
Wavetable Synthesis
Wavetable Synthesis is used in digital musical instruments to produce natural tone-like sounds.
The wavetable is a section of memory that contains a list of values corresponding to the desired waveform.
The computer reads the numbers from the list at a steady rate (the sampling rate), repeating the table when the end is reached.
If the table contains a single cycle of the waveform, the frequency produced would simply be the sample rate divided by the number of values in the table.
The sound of an existing instrument (a single tone) is sampled and parsed into a sequence of wavetables having one period or cycle in each wavetable.
Upon playback, these wavetables are used for sample “lookup”.
The output waveform is not normally static and evolves slowly in time as one wavetable is mixed with another, creating a changing waveform.
Looping occurs when the wavetable is halted, slowed or reversed in time.
Download Image For Larger View
| purewavetablesynth_l.jpg |
Sample Based Synthesis
Multiple samples are taken of the various idiomatic components of a sound.
The waveforms are sampled sounds and instruments instead of fundamental wave forms such as the sine wave.
These sounds are played back at appropriate times depending on the performance input resulting in very authentic recreation of the initial sound.
Physical Modeling
Uses a set o equations and algorithms to simulate a physical source of sound.
Complete Lesson 9 Activities #1 - 5
Complete Lesson 9 Workbook Assignment
Complete Lesson 9 Virtual Inst. & Synthesis Quiz